Since this is my first year teaching third grade, this was my first time teaching the three properties of addition- commutative, associative, and identity. I knew I was going to bore my kids (and myself!) if I just stated the properties and expected them to remember the meaning of each one. To make it a little more engaging, I used a pan balance, unifix cubes, and hand motions to explain each of the three properties.
First up was the Commutative Property of Addition. We discussed the base word in commutative- commute. I explained that my commute to work is the drive back and forth. To help remember that commute meant back and forth, we used the hand motion below:
After we practiced the hand motion a few times, I used unifix cubes to model how the Commutative Property works. On one side of the pan balance, I placed 3 purple cubes added to 5 black cubes (to represent 3 + 5). On the right side of the pan balance, I placed 5 black cubes added to 3 purple cubes (to represent 5 + 3).
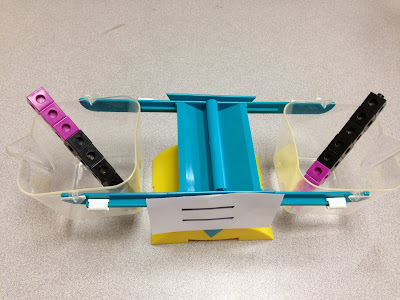 |
| 3 + 5 = 5 + 3 |
For the Associative Property of Addition, we also discussed the base word in associative- associate. I explained that associate means to put things together or to group them. I showed them an equation with parentheses and we talked about how the parentheses group numbers together and tell you to add them first. To remember the meaning of associate, we used the hand motion below:
Next, I used the pan balance and unifix cubes to model how the Associate Property works. On the left side of the balance, I placed 3 purple cubes on top of 2 green cubes next to 5 black cubes. On the right side, I placed 3 purple cubes next to 2 green cubes on top of 5 black cubes. This represented the equation ( 3 + 2 ) + 5 = 3 + (2 + 5).
 |
( 3 + 2 ) + 5 = 3 + ( 2 + 5 )
|
I again asked the kids which side had more. They agreed that both sides were the same and drew their own conclusion that it doesn't matter how you group numbers together in an addition equation, the answer will still be the same.
Last up was the Identify Property of Addition. This is by far the easiest to understand and model! The hand motion that we used for the identify property is below:
This helped the kids remember that the Identify Property of Addition means "zero change" to the number. To model this property, I placed 6 purple cubes on the left side of the pan balance. I then demonstrated adding zero more (I used my hands to show that I wasn't adding any more!) I asked them which number should go on the right side of the pan balance to make both sides of the equation the same. They told me that I needed to add 6 purple cubes since the number didn't change. They drew the conclusion that any time you add 0 to a number, the number stays the same.
I taught this lesson yesterday in math and I was totally shocked to see that they remembered the meaning of each Property of Addition today! They were so excited to show me the hand motions and explain the rule for each one. I really think that using hand motions helps the kids to remember the meaning of each property, which in turn helps them apply it to a math equation.
On a side note- I really like using the pan balance to demonstrate addition and subtraction problems. All too often, kids assume that one side of the equal sign is the "answer". The pan balance, with a notecard taped to the middle, helps them understand that the equal sign really means "the same as." The first time that they see an equation such as 4 + 5 = 12 - 3, they are totally thrown off! The balance is a great visual to help them understand!





This is just what I need. What creative ideas you have! Thank you for sharing!
ReplyDeleteLaura Santos
Core Inspiration by Laura Santos
My 1st grade kid is struggling with "balancing the equation". I was about to give up and go to a learning store to buy a balance pan but this online tool would fill the gap. I still would like to at least cobble together a cheapo homemade balance pan for a real-world "grasp" of it. Thanks!
ReplyDeletethis in an excellent explanation!
ReplyDeleteI want to thank Emu Temple for the accurate readings he gave me and for also helping me bring back my lover.. If you are here and you are having any relationship problem please Contact Emu Temple on Via Email: emutemple@gmail.com OR Via WhatsApp +2347012841542 and result is 100% guarantee and He do lot more as:*Love Spells*Lost Love Spells*Divorce Spells*Stop Divorce Spell*Marriage Spells*Binding Spells*Breakup Spells*You want to be promoted in your office*Want to satisfy your lover*Death Spell*Gay/Lesbian Love Spell*Court case Spell*Politics Spell*Good-luck Spell*Bad Luck Remover Spell*Do as i say Spell*General cleansing Spell*Herbal remedy for all kind of disease
ReplyDelete*Lottery Spell*Pregnancy Spell*Peins enlargement
I want to thank Emu Temple for the accurate readings he gave me and for also helping me bring back my lover.. If you are here and you are having any relationship problem please Contact Emu Temple on Via Email: emutemple@gmail.com OR Via WhatsApp +2347012841542 and result is 100% guarantee and He do lot more as:*Love Spells*Lost Love Spells*Divorce Spells*Stop Divorce Spell*Marriage Spells*Binding Spells*Breakup Spells*You want to be promoted in your office*Want to satisfy your lover*Death Spell*Gay/Lesbian Love Spell*Court case Spell*Politics Spell*Good-luck Spell*Bad Luck Remover Spell*Do as i say Spell*General cleansing Spell*Herbal remedy for all kind of disease
*Lottery Spell*Pregnancy Spell*Peins enlargement
My ex just returned like you assured me. Thank you Dr Tino My ex is back, thank you so much!!! I saw a comment online about how Dr Tino help someone brought back his wife who left for weeks.I contacted Dr Tino, he told me what to do and I did according to his instructions. That was it, everything changed all of a sudden. Now I am happy again with my husband, You can contact him via WhatsApp +1(813) 360 1365 or Mail him @ (palaceofsolutions@gmail . Com)
ReplyDelete